Skolem , Thoralf Albert

(1887–1963) Norwegian mathematician

The son of a teacher, Skolem was born at Sandsvaer in Norway and educated at the University of Oslo. He joined the faculty in 1911 and was appointed professor of mathematics in 1938, a post he held until his retirement in 1950.

Skolem is best known for his work in mathematical logic, including his contribution to the proof of the Lowenheim-Skolem theorem and the construction of the Skolem paradox.

The Lowenheim theorem of 1915, as generalized by Skolem in 1920, simply states that any schema satisfiable in some domain is satisfiable in a denumerably infinite domain. Denunerably infinite domains, also known as countable domains, can be matched in a one–one correspondence with the domain of natural numbers. Clearly the natural numbers are denumerably infinite, as are the even numbers and the rational numbers. There are in fact just as many even numbers as natural numbers, as can be seen from the correspondences set out below:

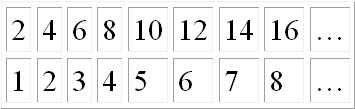There are, however, domains, such as the domain of the real numbers, which cannot be put into such a correspondence with the natural numbers. Such domains are nondenumerably infinite.

Skolem pointed out in 1922 that this result leads to a new paradox in set theory. There are sets, the set of real numbers for example, which are nondenumerable. Yet, by the Lowenheim-Skolem theorem, they must be satisfiable in a denumerably infinite domain. Skolem proposed to defuse the paradox by claiming that notions such as nondenumerability have no absolute meaning, but can only be understood within the confines a particular axiomatic system. Thus a set may be nondenumerable within a system and denumerable outside. Consequently, the paradox will fail to arise.